×

Courses

Chapters

>
<

Aptitude

Area

What does area mean ?
  • Area is nothing but the amount of space taken up by a shape which has a closed boundary.
  • In geometry, the area can be defined as the space occupied by a flat shape or the surface of an object. The area of a figure is the number of unit squares that covers the surface of that closed figure.
  • Area is measured in square units such as square centimteres, square feet, square inches, etc.
Why Area is so Important ?

  • In general it messured at the time of any kind of construction or renovation.
  • Adding to that the use of area has many practical applications in
    1. Architecture
    2. Building
    3. Farming
    4. Science
    5. and concluding which things can fit in your floor !

Then how do we gonna messure it ?

  • The basic unit of area in the metric system is the square meter, in which is a square has 1 meter on each sides.
  • So in other systems area can also be messured in "square mm", "square cm" etc.
  • The area can be messured in different way such as
    1. by using formulas
    2. counting squares
    3. adding up different shapes
    4. by coordinates.

Area of Rectangle

What is area of Rectangle ?

  • Area occupied by a rectangle within its boundary is called the area of the rectangle.
  • Let us draw unit squares inside the rectangle. Each unit square is a square of length 1 inch.

    Now, let's count the number of unit squares in the figure given. How many squares can you see ?


    There are 15 squares in total.
    We have already learned that area is measured in square units.
    Since the unit of this rectangle is "inches," the area is measured and written in square inches.
    Thus, the area of the rectangle = 15 square inches.

How do we find the area of a rectangle ?

  • The area of a rectangle is equal to its length times its width.
  • The steps to find the area of a rectangle:
    1. Note the dimensions of length and breadth from the given data.
    2. Find the product of length and breadth values.
    3. Write the answer in square units.

Diagonal of a Rectangle

  • The diagonal of a rectangle is the straight line inside the rectangle connecting its opposite vertices.
  • We can find the length of the diagonal by using the Pythagoras theorem.
  • The area can be messured in different way such as
    1. $(Diagonal)^2 = (Length)^2+(Breadth)^2$
    2. $(Length)^2 = (Diagonal)^2-(Breadth)^2$
    3. $Length = \sqrt{(Diagonal)^2-(Breadth)^2} $

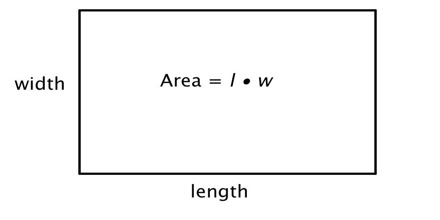
      4. Now, the formula to calculate the area of a rectangle is $Length$ × $Breadth$. Alternatively, we can write this formula as $\sqrt{(Diagonal)^2-(Breadth)^2} $.

      So, Area of a Rectangle $= Breadth$ x $\sqrt{(Diagonal)^2-(Breadth)^2} $.

Area of Square

What is Area of Square ?

  • The area of a square is defined as the number of square units needed to fill that square.
  • It is a quadrilateral with the following properties.
    1. The opposite sides are parallel.
    2. All four sides are equal.
    3. All angles measure 90º.

Calculating Area of Square

  • Algebraically, the area of a square can be found by squaring the number representing the measure of the side of the square.

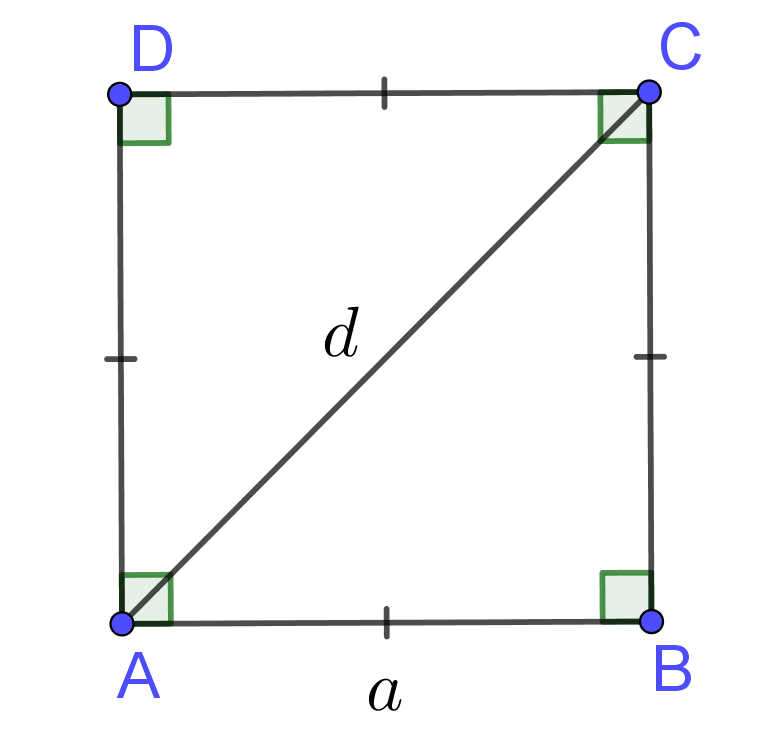
  • The formula for the area of a square when the sides are given is:

      Area of a square = $Side$ × $Side$ = $a^2$

Area from Diagonal of the Square

  • The area of a square can also be found with the help of the diagonal of the square.
  • Let us understand the derivation of this formula with the help of the following figure, where $d$ is the diagonal and $a$ represents the sides of the square.
  • Here the side of the square is $a$ and the diagonal of the square is $d$.
  • Applying the Pythagoras theorem we have $d^2 = a^2 + a^2$; $d^2 = 2a^2$; $d = \sqrt{2}a$; $a = \frac{d}{\sqrt{2}}$.
  • Now, this formula will help us to find the area of the square, using the diagonal. Area = $a^2$ = $(\frac{d}{\sqrt{2}})^2 = \frac{d^2}{2}$.

    Therefore, the area of the square is equal to $\frac{d^2}{2}$.

Area of Triangle

What is area of Triangle ?

  • The area of a triangle is defined as the total space occupied by the three sides of a triangle in a 2-dimensional plane.
  • The area of a triangle is calculated in different ways depending on the type of triangle and the situation given.
  • A triangle can be of these types:
    1. Isosceles Triangle
    2. Equilateral Triangle
    3. Scalene Triangle
    4. Acute Triangle
    5. Obtuse Triangle
    6. Right Angle Triangle

Area of Triangle Using Heron's Formula

  • Heron's formula is used to find the area of a triangle when the length of the 3 sides of the triangle is known. To use this formula, we need to know the perimeter of the triangle which is the distance covered around the triangle and is calculated by adding the length of all three sides.
  • Heron’s formula has two important steps.
    1. Step 1: Find the semi perimeter (half perimeter) of the given triangle by adding all three sides and dividing it by 2.
    2. Step 2: Apply the value of the semi-perimeter of the triangle in the main formula called 'Heron’s Formula'.
  • Consider the triangle ABC with side lengths a, b, and c. To find the area of the triangle we use Heron's formula:

Area of Triangle With 2 Sides and Included Angle (SAS)

  • When two sides and the included angle by the giv en two sides of a triangle are given, we can use a formula that has three variations according to the given dimensions.
  • When sides $b$ and $c$ and included angle $A$ is known, the area of the triangle is:
    1. Area (∆ABC) = $\frac{1}{2}$ × bc × $sin(A)$
  • When sides $a$ and $b$ and included angle $C$ is known, the area of the triangle is:
    1. Area (∆ABC) = $\frac{1}{2}$ × ab × $sin(C)$
  • When sides $a$ and $c$ and included angle B is known, the area of the triangle is:
    1. Area (∆ABC) = $\frac{1}{2}$ × ac × $sin(B)$

Area of an Equilateral Triangle

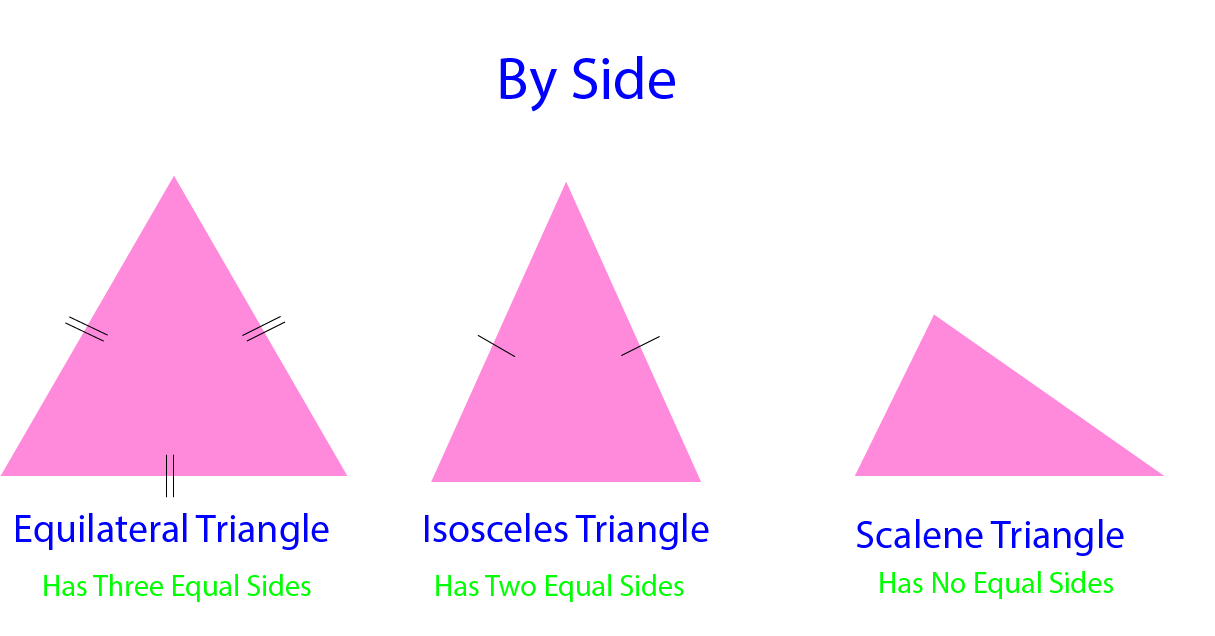
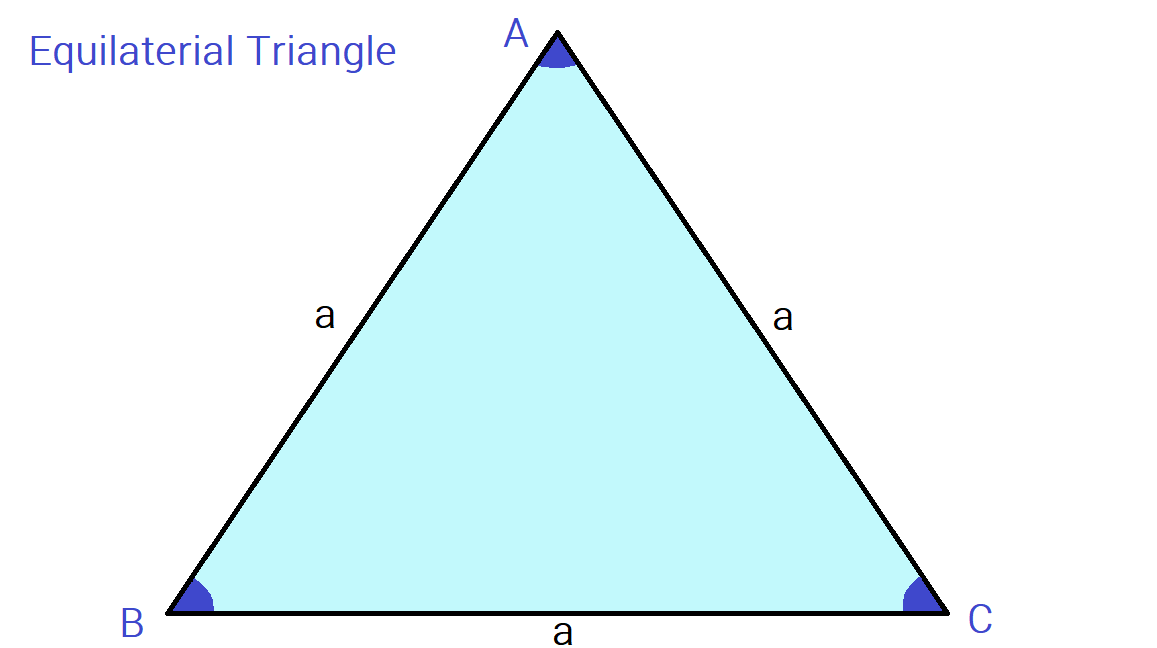
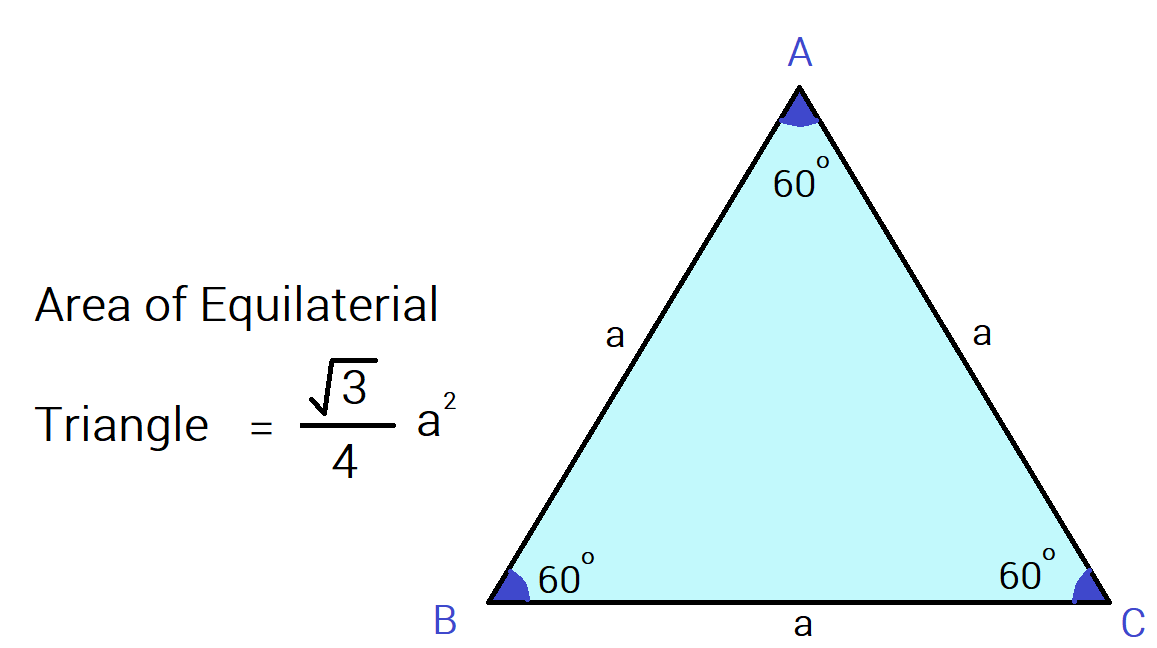
  • An equilateral triangle is a triangle where all the sides are equal. The perpendicular drawn from the vertex of the triangle to the base divides the base into two equal parts.

  • To calculate the area of the equilateral triangle, we need to know the measurement of its sides.

  • Area of an Equilateral Triangle = $A = \frac{\sqrt{3}}{4} × side^2$

Area of a Right-Angled Triangle

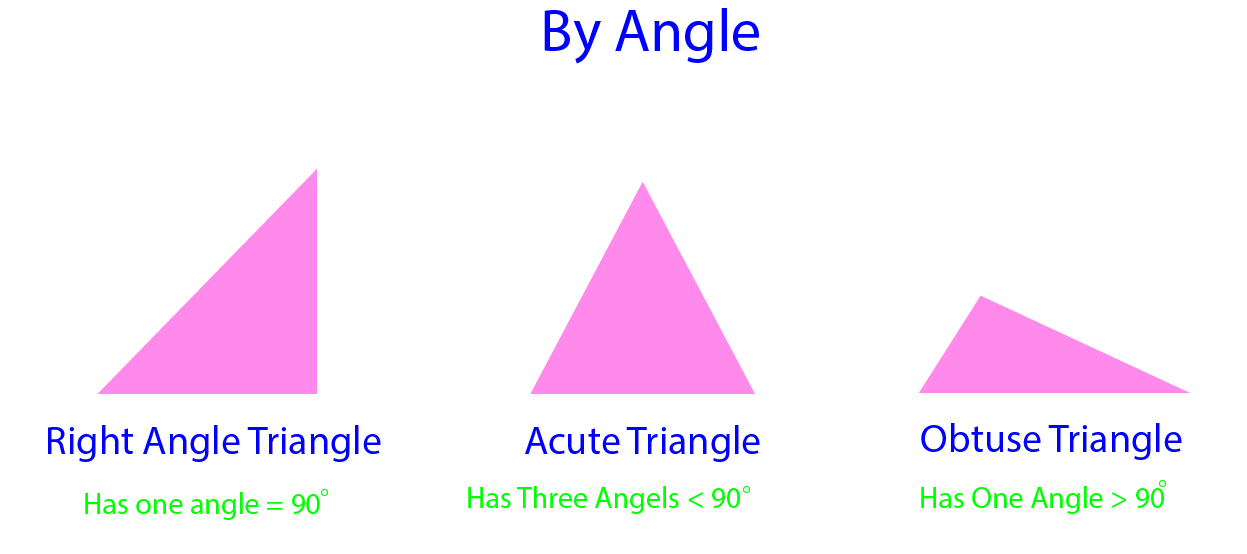
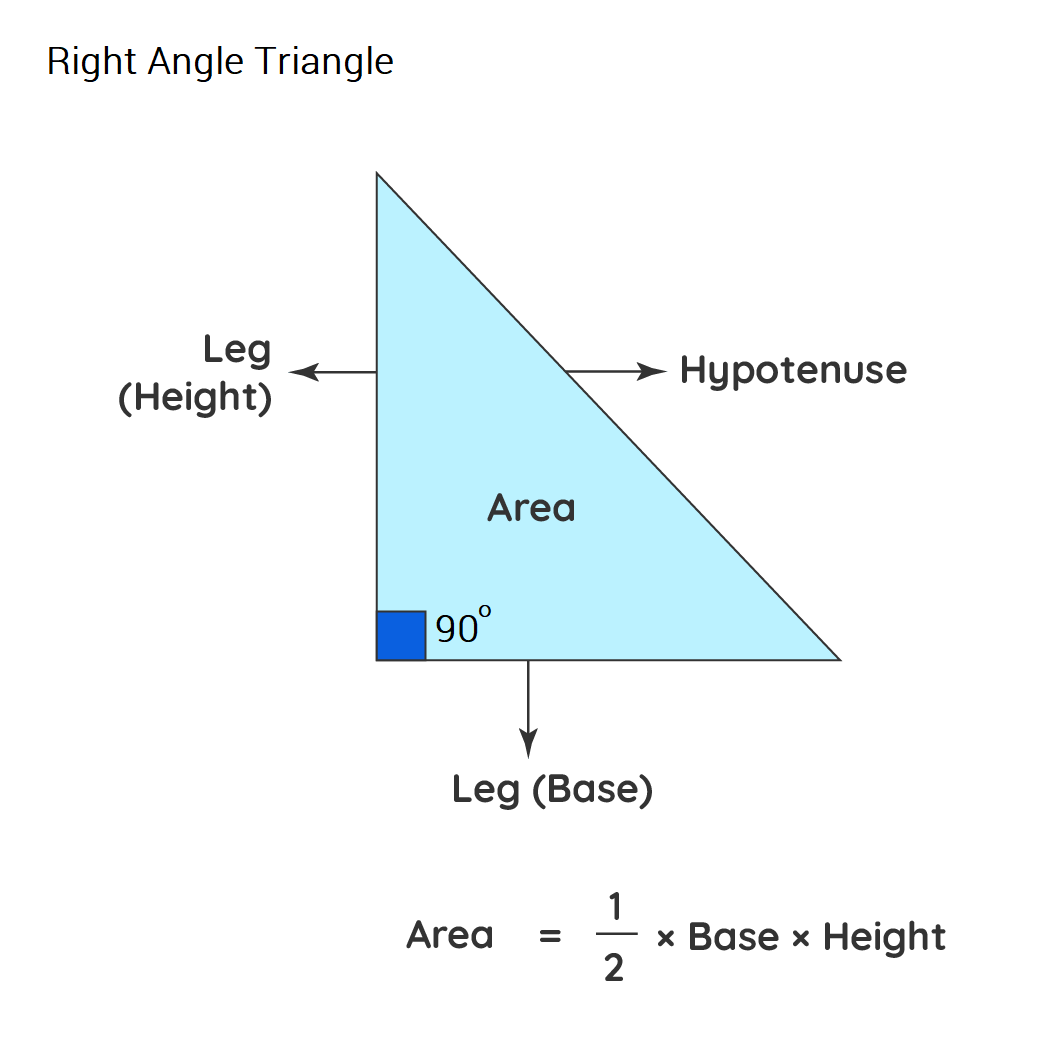
  • A right-angled triangle, also called a right triangle, has one angle equal to 90° and the other two acute angles sum up to 90°.

  • Therefore, the height of the triangle is the length of the perpendicular side.

  • Area of a Right Triangle = A = $\frac{1}{2}$ × Base × Height

Area of an Isosceles Triangle

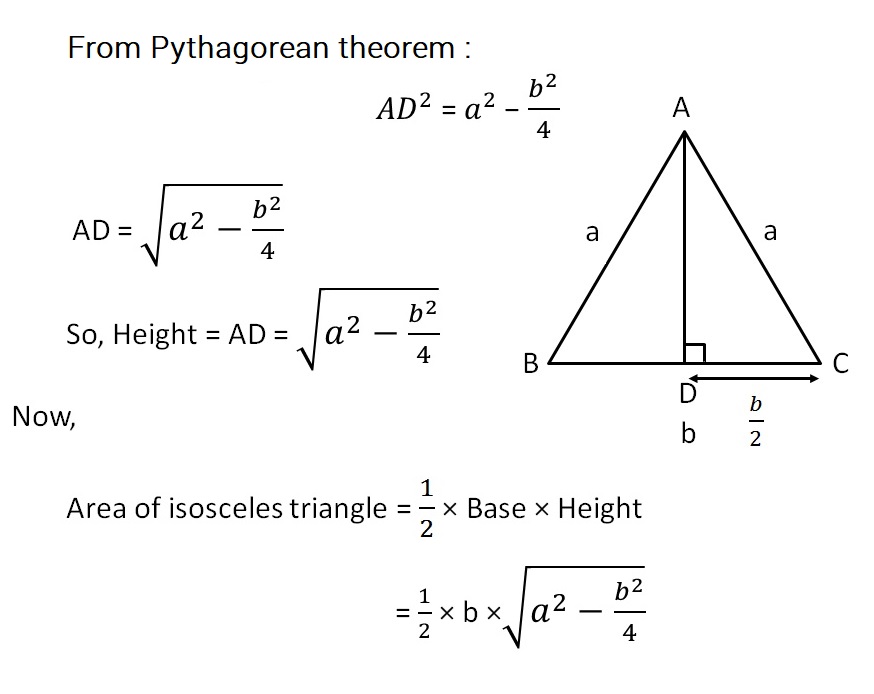
  • An isosceles triangle has two of its sides equal and the angles opposite the equal sides are also equal.

  • Area of an Isosceles Triangle = A = $\frac{1}{4}b\sqrt{4a^2−b^2}$
    where $b$ is the base and $a$ is the measure of one of the equal sides.

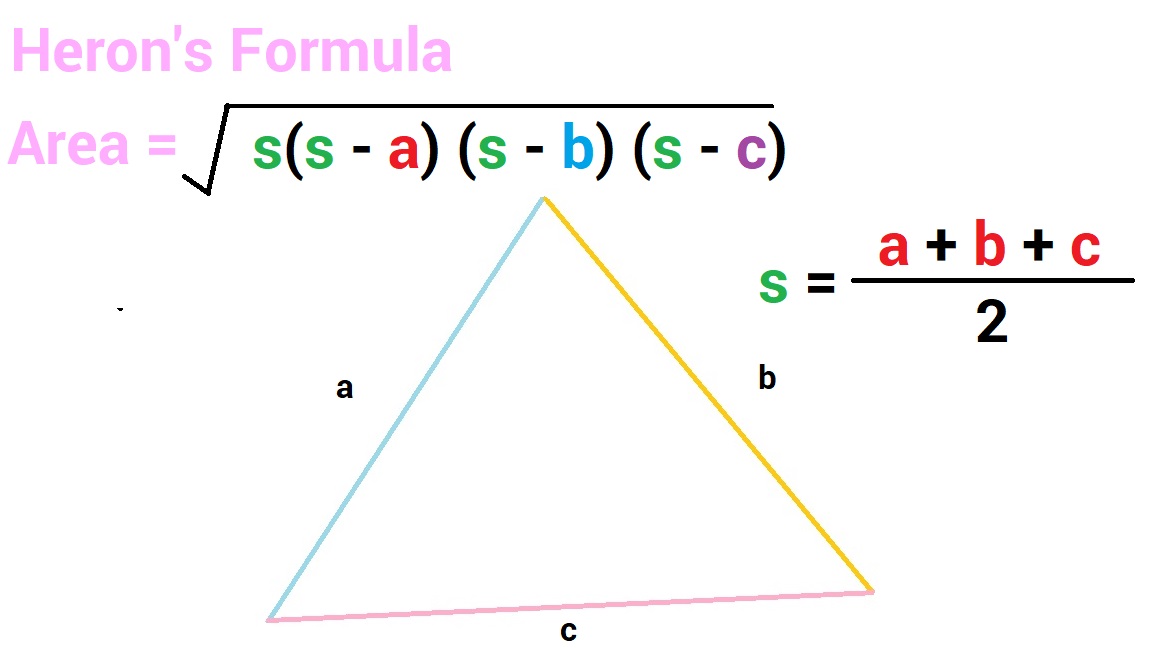
  • Observe the table given below which summarizes all the formulas for the area of a triangle.

Area of Trapezium

What is Area of Trapezium ?

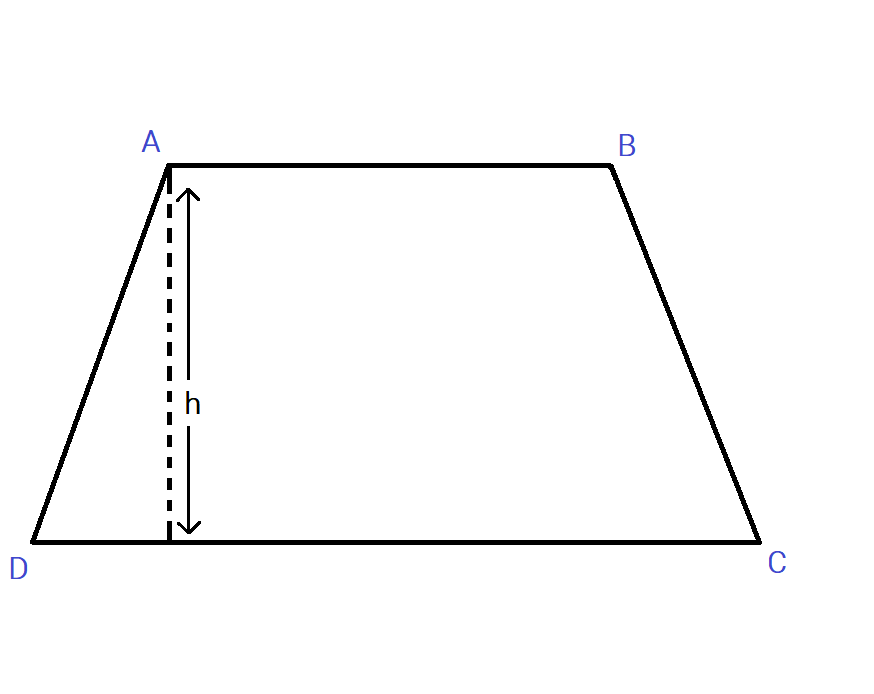
  • A trapezium is a type of quadrilateral that has one pair of parallel sides(generally referred to as bases).

  • The other pair of sides of a trapezium can be non-parallel and are known as legs.

  • The area of a trapezium is the total space covered by a trapezium in a two-dimensional plane.

Derivation of Area of Trapezium
The steps given below can be followed to find the area of a trapezium:

    1. Note the dimensions of the lengths of the parallel sides (bases) of the trapezium from the given data.
    2. Calculate the sum of the bases.
    3. Multiply the value of the sum of bases by height or altitude of the trapezium and by $\frac{1}{2}$.
    4. Write the answer in square units.

Derivation of Area of Trapezium Formula Using a Parallelogram

  • The area of a trapezium can be calculated using the lengths of two of its parallel sides and the distance (height) between them.
  • The formula to calculate the area (A) of a trapezium using base and height is given as,A = $\frac{1}{2}(a + b)h$ where,
    1. a and b = bases of trapezium, and,
    2. h = height (the perpendicular distance between a and b)

Derivation of Area of Trapezium Formula Using a Parallelogram

  • To derive the formula for the area of a trapezium using parallelogram, we will consider two identical trapeziums, each with bases a and b and height h.
  • Let A be the area of each trapezium. Assume that the second trapezium is turned upside down as shown in the figure below.
  • We can see that the new figure obtained by joining the two trapeziums is a parallelogram whose base is a + b and whose height is h.
  • We know that the area of a parallelogram is base × height. The area of the above parallelogram is, A + A = 2A.
  • Thus, $2A = (a + b) h ⇒ A = (a+b)\frac{h}{2}$

Area of Rhombus

What is Area of a Rhombus?

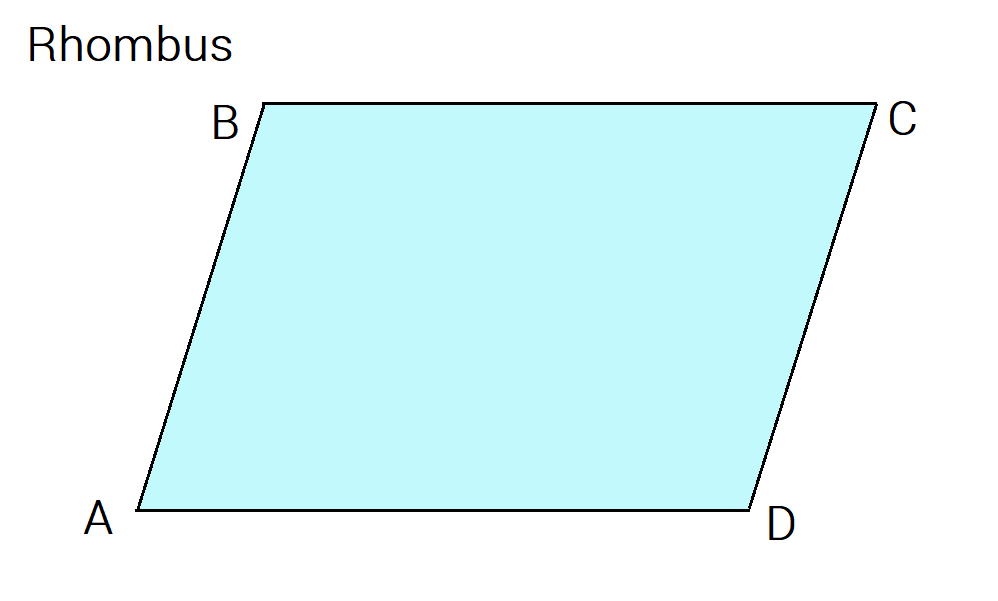
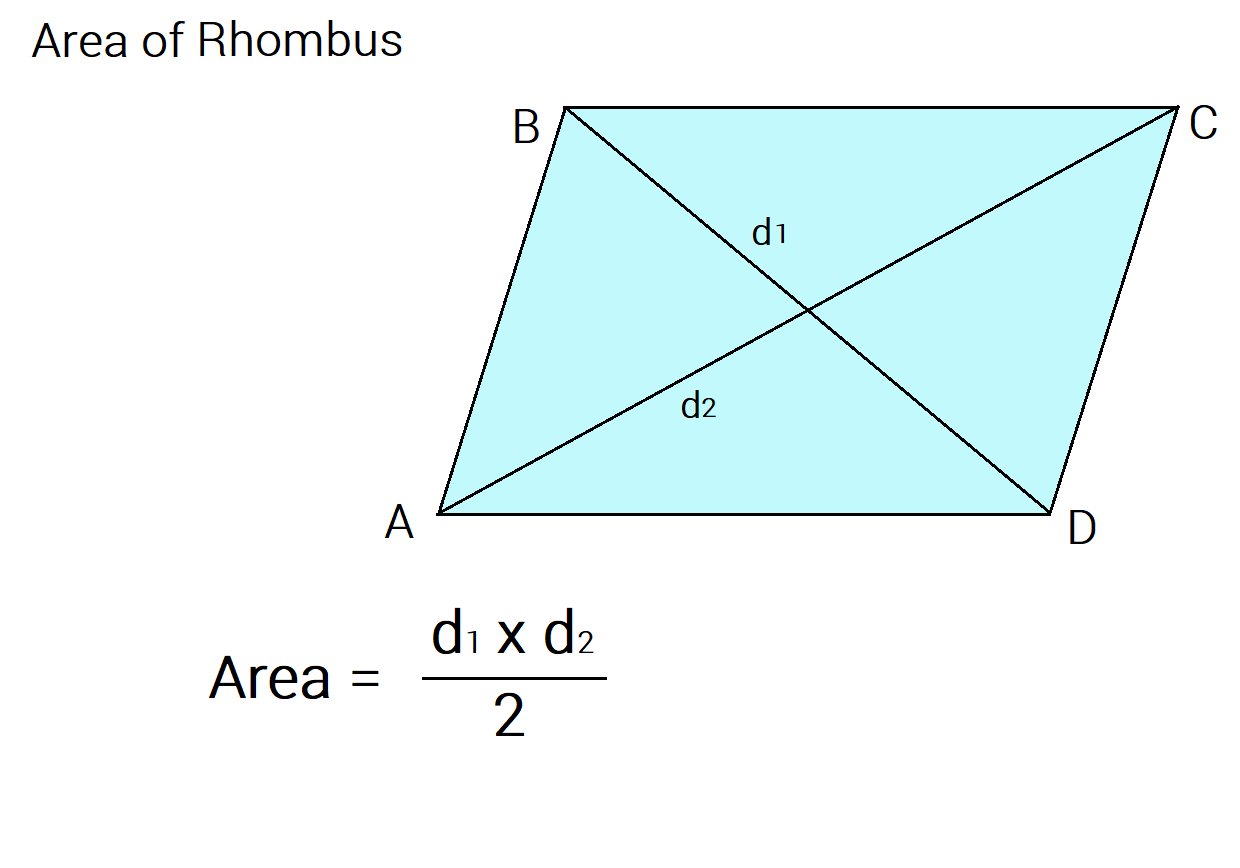
  • The area of a rhombus can be defined as the amount of space enclosed by a rhombus in a two-dimensional space.

  • Rhombus is a parallelogram with the opposite sides parallel, the opposite angles equal, and the adjacent angles supplementary.

  • The following properties are used to define a rhombus.

    1. A rhombus is an equilateral quadrilateral because all the sides have equal lengths
    2. In a rhombus, diagonals bisect each other at right angles.
    3. The diagonals are angle bisectors.
    4. The area of a rhombus can be found in different ways: using base and height, using diagonals, and using trigonometry.

Finding the area of Rhombus
Formula for Area of Rhombus When Base and Height Are Known

  • Different formulas can be used to calculate the area of a rhombus depending upon the parameters known to us.
  • The different formulas followed for the calculation of the area of a rhombus are,
    1. Using base and height
    2. Using diagonals
    3. Using trigonometry

Formula for Area of Rhombus When Base and Height Are Known

  • The area of a rhombus is equal to half the product of the lengths of the diagonals.
  • The formula to calculate the area of a rhombus using diagonals is given as,
  • Area = $\frac{(d_{1} × d_{2})}{2}$ sq. units, where, d1 and d2 are the diagonals of the rhombus.

Formula for Area of Rhombus When Side and Angles Are Known

  • We apply the concept of trigonometry while calculating the area when sides and angles are known.
  • We can use any angle because either the angles are equal or they are supplementary, and supplementary angles have the same sine.
  • Area of a Rhombus = $side^2 × sin(A)$ sq. units, where $A$ is an interior angle.

Area of Parallelogram

What Is the Area of Parallelogram?

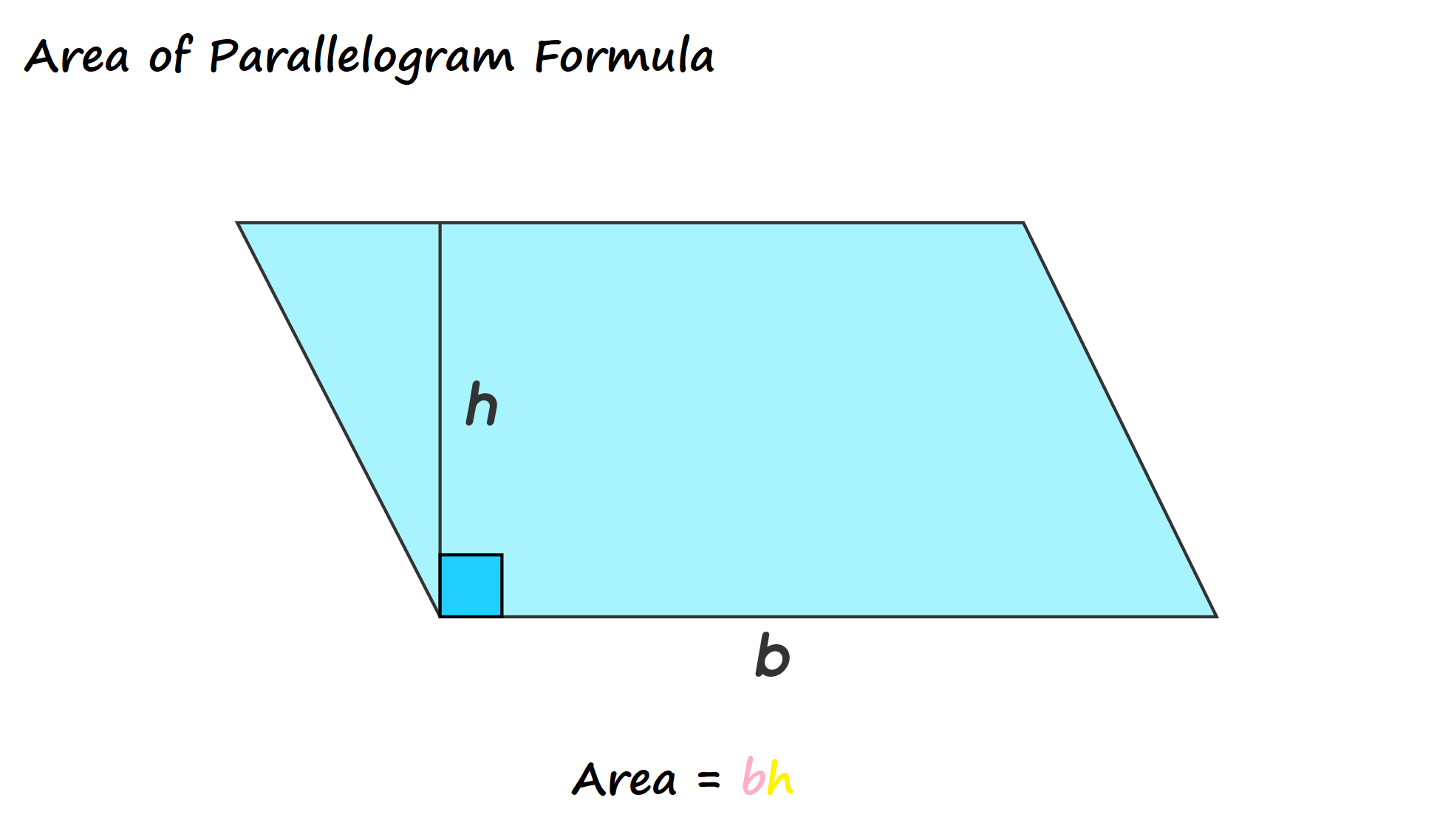
  • It is the region enclosed or encompassed by a parallelogram in two-dimensional space.

  • A parallelogram is a four-sided, 2-dimensional figure with:

    1. two equal, opposite sides,
    2. two intersecting and non-equal diagonals, and
    3. opposite angles that are equal

Area of a Parallelogram Formula

  • The area of a parallelogram can be calculated by multiplying its base with the altitude
  • The base and altitude of a parallelogram are perpendicular to each other as shown in the following figure.
  • Area of parallelogram = $b$ × $h$ square units where,
    1. b is the length of the base
    2. h is the height or altitude

Parallelogram Area Using Height

  • Suppose $a$ and $b$ are the set of parallel sides of a parallelogram and $h$ is the height (which is the perpendicular distance between $a$ and $b$).
  • Then the area of a parallelogram is given by:
    1. Area = Base × Height
    2. A = b × h [square units]

Parallelogram Area Using Lengths of Sides

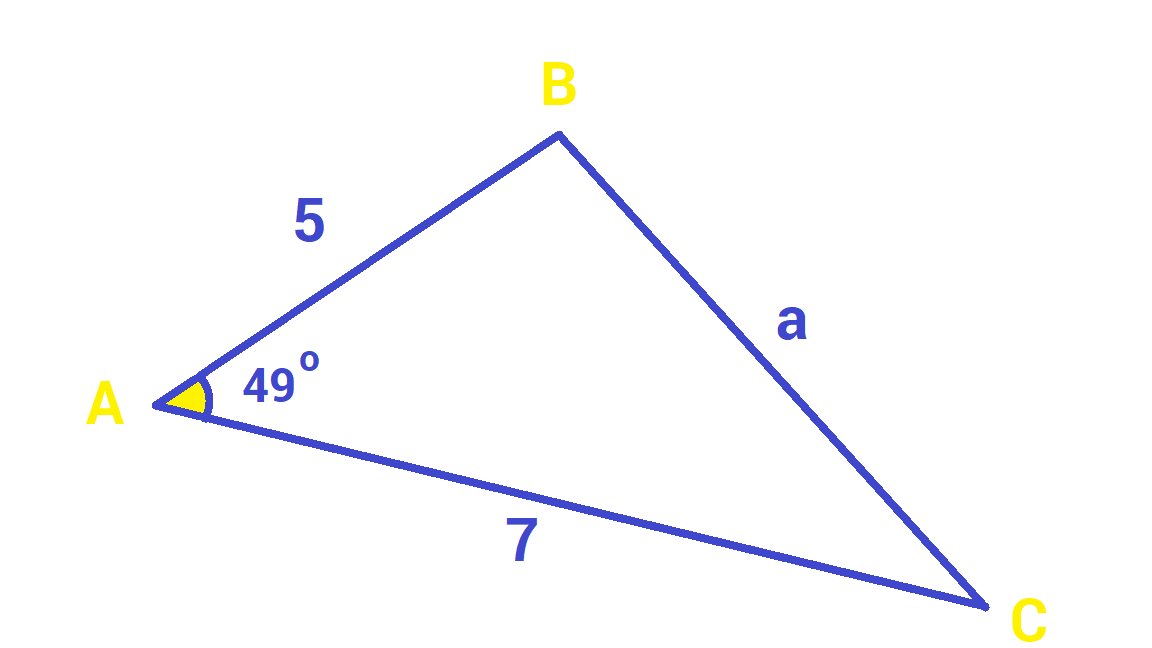
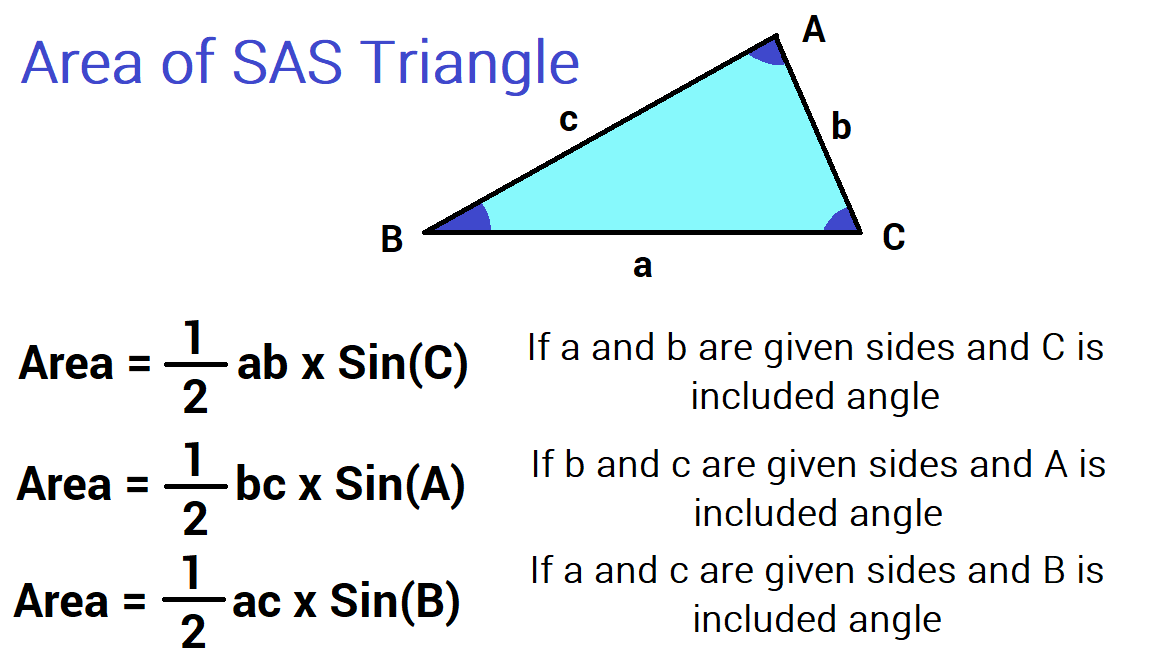
  • The area of a parallelogram can also be calculated without the height if the length of adjacent sides and angle between them are known to us.

  • We can simply use the area of the triangle formula from the trigonometry concept for this case.

  • Area = ab sin (θ)
    where,

    1. a and b = length of parallel sides, and,
    2. θ = angle between the sides of the parallelogram.

Parallelogram Area Using Diagonals

  • The area of any given parallelogram can also be calculated using the length of its diagonals.
  • There are two diagonals for a parallelogram, intersecting each other at certain angles.
  • Suppose, this angle is given by x, then the area of the parallelogram is given by:
    Area = $\frac{1}{2}$× $d_{1}$ × $d_{2}$ $sin(X)$, where,
    1. $d_{1}$ and $d_{2}$ = Length of diagonals of the parallelogram, and
    2. x = Angle between the diagonals.

Area of Circle

What is Area of Circle ?

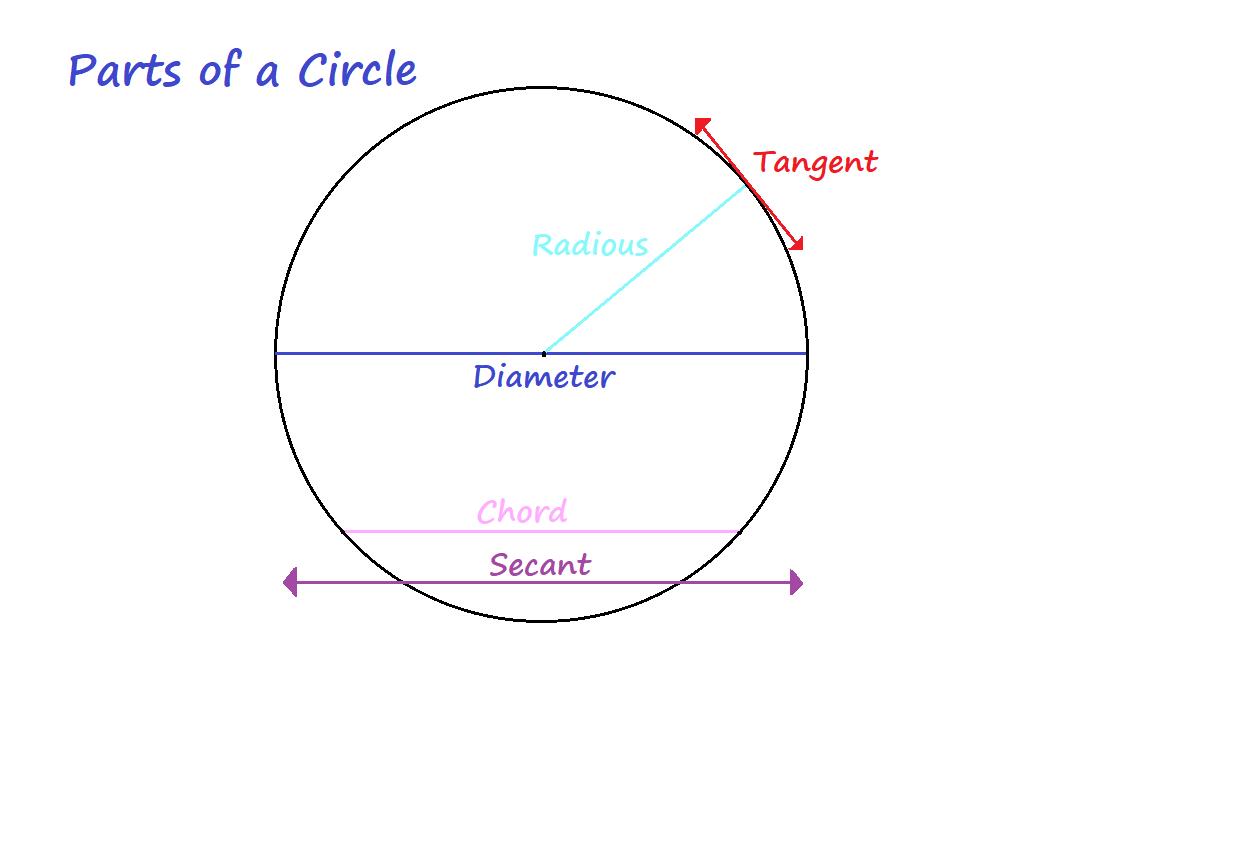
  • The area of a circle is the space occupied by the circle in a two-dimensional plane.

  • A circle is a collection of points that are at a fixed distance from the center of the circle.

  • For a circle with radius $r$ and circumference $C$:

    1. π = Circumference/Diameter
    2. π = $\frac{C}{2r}$ = $\frac{C}{d}$
    3. C = 2πr

Parts of a circle

  • Different parts of Circle :

    1. Centre
    2. Circumference
    3. Chord
    4. Radius
    5. Diameter
    6. Minor segment
    7. Major segment
    8. Interior part of the circle
    9. Exterior part of the circle
    10. Arc

Area of Circle Formulas
Area of Square by Diagonals.

  • The area of a circle can be calculated in intermediate steps from the diameter, and the circumference of a circle. From the diameter and the circumference, we can find the radius and then find the area of a circle.
  • But in Simple ways Area of a circle can be calculated by using the formulas:
    1. Area $= π × r^2$, where $r$ is the radius.
    2. Area = $\frac{π}{4}$ × $d^2$, where $d$ is the diameter.
    3. Area = $\frac{C^2}{4π}$, where $C$ is the circumference.

Area of Ellipse

What is Ellipse ?

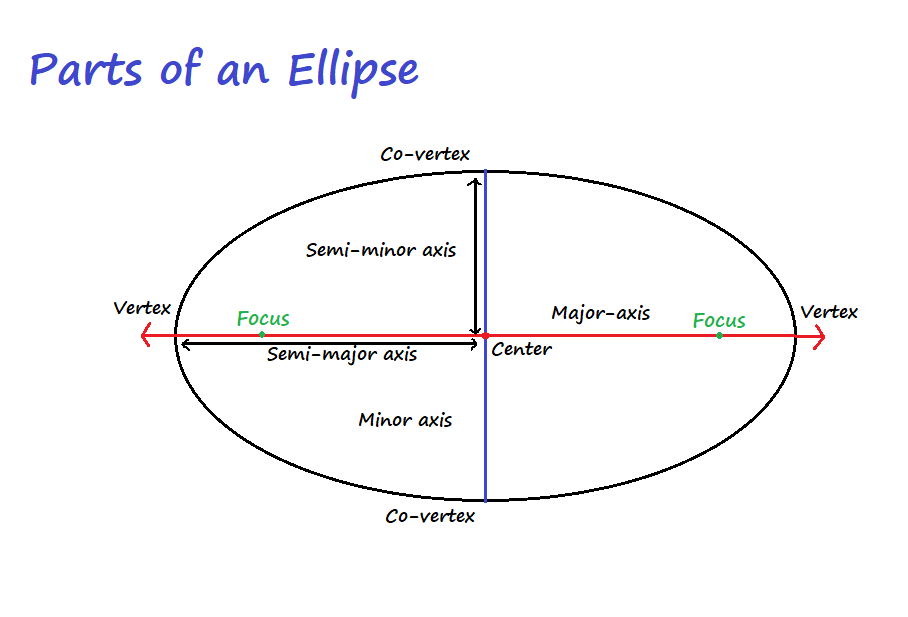
  • Ellipse is a 2-D shape obtained by connecting all the points which are at a constant distance from the two fixed points on the plane..

  • he fixed points are called foci. F1 and F2 are the two foci.

  • As an ellipse is not a perfect circle, the distance from the center of the ellipse to the points on the circumference is not constant.So, an ellipse has two radii.

    1. The longest chord in the ellipse is called the major axis of the ellipse.
    2. The minor axis is the chord that is the perpendicular bisector to the major axis.

How to find Area of Ellipse ?

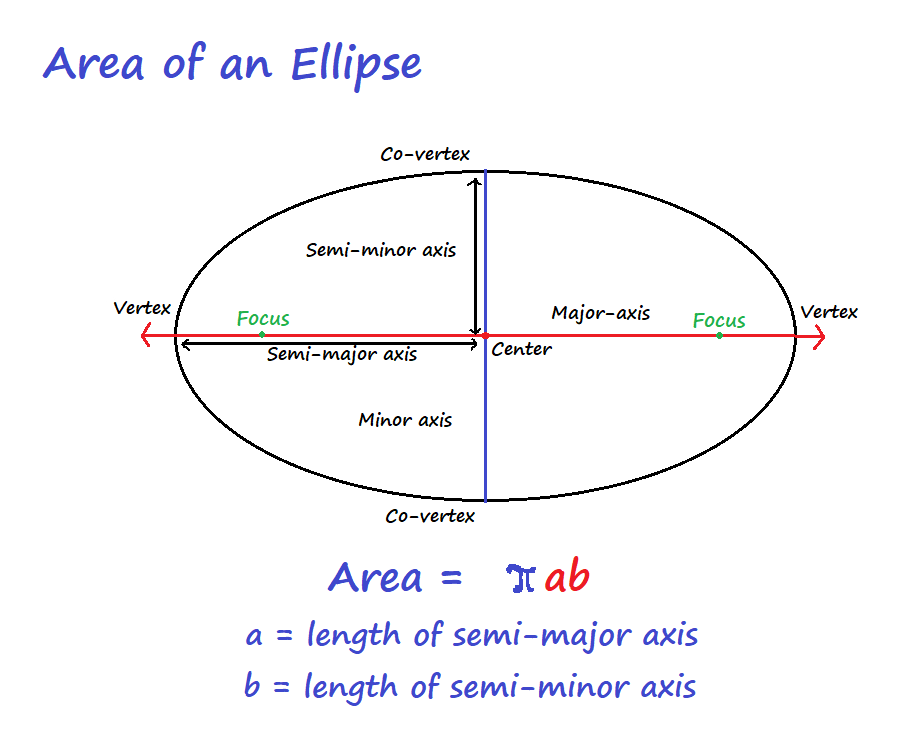
  • We can calculate the area of an ellipse using a general formula, given the lengths of the major and minor axis.

  • The formula to find the area of an ellipse is given by,
    Area of ellipse = π a b
    where,

    1. a = length of semi-major axis
    2. b = length of semi-minor axis

→
←