Aptitude
Introduction to Ratio and Propertion
Ratio
In some situations, the comparison by a division process makes good sense when compared to performing their difference. On this page you’ll learn How to Solve Ratio And Proportion Quickly.
Therefore, the comparison of two quantities by the process of division method is called as ‘Ratio’ between two numbers. The two numbers in a ratio can only be compared when they have the same unit. We make use of ratios to compare two things. The sign used to denote a ratio is ‘:’.
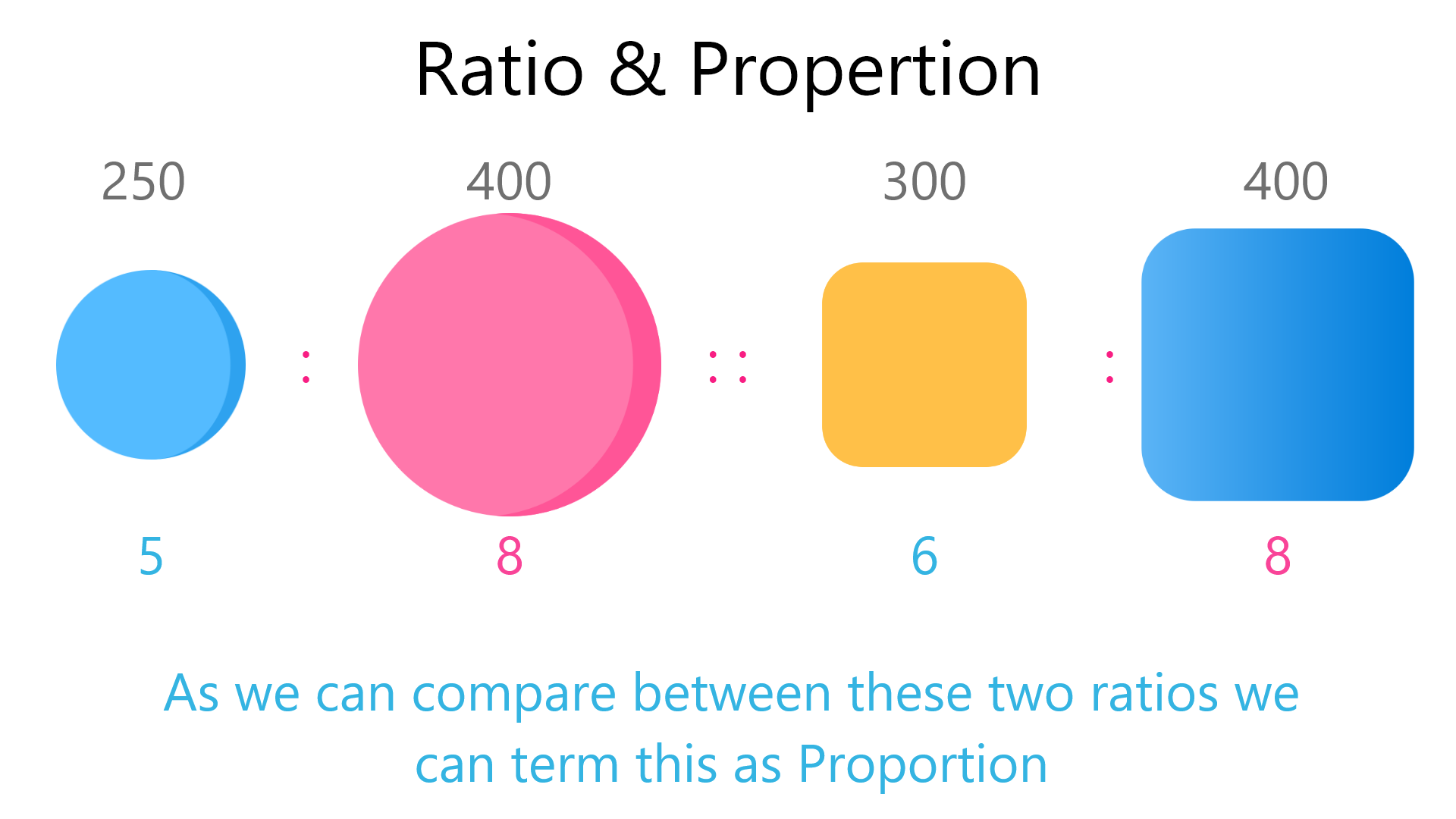
Proportion
Ratio and proportions are said to be faces of the same coin. When two ratios are equal in value, then they are said to be in proportion. In simple words, it compares two ratios. Proportions are denoted by the symbol ‘::’ or ‘=’.
1.Find the compound ratio of (9 : 10), (11 : 13), (15 : 19).
Options:
A. 156:157
B. 297:494
C. 221:431
D. 1:5
Solution:
If we compound two or more ratio, then, a : b and c : d will become ac:bd.
Therefore,$ (9 : 10), (11 : 13), (15 : 19) = \frac{9}{10} \times \frac{11}{13} \times \frac{15}{19} = \frac{1485}{2470} $
$ = \frac{297}{494}$
Correct Option : B
2. Find the composite ratio of 2 : 3, 3 : 5, 5 : 7.
Options:
A. 11 : 24
A. 11 : 24
C. 14 : 23
D. 24 : 97
Solution:
The composite ratio is intended by $ \frac{2}{3} \times frac{3}{5} \times \frac{5}{7} = frac{30}{105} = \frac{2}{7}
Correct Option: B
3. Find the compounded ratio of (3 : 7), (9:5), and (11:21)
Options:
A. 99:245
B. 13:17
C. 114:221
D. 305:711
Solution:
If we compound two or more ratio, then, a : b and c : d will become ac:bd.
Therefore, (3 : 7), (9:5), and (11:21)= $ \frac{3}{7} \times \frac{9}{5} \times \frac{11}{21} = \fraC{297}{735} = \frac{99}{245}
Correct Option: A
1.Rs. 27440 is divided among 5 females, 3 men, and 1 child. The ratio of each woman, man, and kid, are 9 : 3 : 2. What is the child’s share?
Options:
A. 550
B. 760
B. 760
D. 952
Solution:
Given, the ratio of females, males, and toddlers = 9 : 3 : 2
No. of females, men, and child are 5, 3, 1
Thus actual ratio of females, men, and child = 9 * 5 : 3 * 3 : 2 * 1 = 45 : 9 : 2
Therefore, part of child = $ (\frac{2}{56}) \times 27440 = Rs. 980$
Correct Option : C
2. An amount of Rs. 58666 is divided among three employees in the ratio of 1/17, 1/19, and 1/21. Find the smallest share.
Options:
A. 17561.7
B. 17200.49
C. 17000
C. 17000
Solution:
Given, three shares $ = \frac{1}{17}, \frac{1}{19}, \frac{1}{21}.$
Therefore, the ratio will be $ \frac{399}{6783}, \frac{357}{6783}, \frac{323}{6783} $
Thus, the ratio is 399 : 357 : 323
The smallest share $ = 58666 \times \frac{323}{1079} $
= 17561.7
Correct Option: A
1.Sahil got old currencies from his cupboard worth Rs. 450 in the denomination of 2 paisa, 5 paisa, and 50 paisa in ratio 5 : 4 : 3. How many 2 paisa coins he got.
Options:
A. 1200
B. 1250
B. 1250
D. 1220
Solution:
Let the number of 2 paisa coins be 5x
Let the number of 5 paisa coins be 4x
Let the number of 50 paisa coins be 3x
Then,$ 2 \times \frac{5x}{100} + 5 \times \frac{4x}{100} + 50 \times \frac{3x}{100} = \frac{180x}{100}$
Given,$ \frac{180x}{100} = 450$
Therefore, $ 450 \times \frac{100}{180} = x $
x = 250
Hence, 2 paisa coins $ = 5 \times 250 = 1250 $
Correct Option : B
2.A person has 180 rupees in the denomination of 5paisa, 10paisa, and 25paisa in ratio 5 : 3 : 1. Calculate how many 25 paisa coins he has.
Options:
A. 225
B. 200
C. 350
D. 300
Solution:
Let the number of 5 paisa coins be 5x
Let the number of 10 paisa coins be 3x
Let the number of 25 paisa coins be x
Then,$ 5 \times \frac{5x}{100} + 10 \times \frac{3x}{100} + \frac{25x}{100} = \frac{80x}{100}$
Given,$ \frac{80x}{100} = 180$
Therefore,$ 180 \times {100}{80} = x$
x = 225
Hence, 25 paisa coins $ = 1 \times 225 = 225 coins $
Correct Option: B
3. A bag contains a certain number of 50 paise coins, 20 paise coins and 10 paise coins inthe ratio 2:3:4. If the total value of all the coins in the bag is Rs.400, find the number of coins of each kind?
Options:
A. 50p coins = 4
20p coins = 6
10p coins = 8
B. 50p coins = 3
20p coins = 7
10p coins = 2
C. 50p coins = 8
20p coins = 4
10p coins = 2
D. 50p coins = 4
20p coins = 5
10p coins = 3
Solution:
Let the number of each coins be 2n , 3n & 4n respectively. According to Question :
⇒ (2n × 50) + (3n × 20) + (4n × 10) = 400
⇒ 100n + 60n + 40n = 400
⇒ 200n = 400
⇒ $ n = \frac{400}{200} $
⇒ n = 2
Now number of each denominations :
⇒ Number of Rs.50 coins = 2n
= 2(2)
= 4
⇒ Number of Rs.20 coins = 3n
= 3(2)
= 6
⇒ Number of Rs.10 coins = 4n
= 4(2)
= 8
Correct Option: A
1.Chetan and Shaheen’s salaries are in the ratio 5 : 9. If both of their salaries are raised by Rs. 4200, then the proportion changes to 22 : 27. Find Shaheen’s salary.
Options:
A. 9250.95
B. 8058.32
C. 7199.97
D. 13580.45
Solution:
Let Chetan and Shaheen’s salaries be 5x and 9x
Given,$ 5x + \frac{4200}{9x} + 4200 = \frac{22}{27} $
$ 135x + 113400 = 198x + 92400 $
63x = 21000
x = 333.33
Therefore, Shaheen’s salary $ = 333.33 \times 9 + 4200 = 7199.97 $
Correct Option : C
2. Salaries of Preeti and Bina are in the ratio 14 : 15. If both get an increment of Rs. 5300, the new ratio becomes 33 : 35. What is Preeti’s salary?
Options:
A. 24000
B. 34980
C. 30100
D. 10200
Solution:
Let Preeti’s salary be 14x, and Bina’s salary be 15x
Given, 14x + 5300 = 33
Given, 15x + 5300 = 35
$ = 14x + \frac{5300}{15x} + 5300 = \frac{33}{35} $
= 490x + 185500 = 495x + 174900
5x = 10600
x = 2120
Therefore, Preeti’s salary $ = 14 \times 2120 + 5300 = 34980 $
Correct Option: B
3.400 g of 25% sugar syrup was added to 600 g of 40% sugar syrup. Find the percentage of the syrup in the mixture.
Options:
A. 22%
B. 34%
C. 31%
D. 38%
Solution:
Amount of sugar syrup in mixture $ 1 = \frac{25}{100} \times 400 = 100 $
Amount of sugar syrup in mixture $ 2 = \frac{40}{100} \times 600 = 240 $
The total amount of sugar syrup = 340
Percentage of sugar syrup in the mixture $ = 340 \times \frac{100}{1000} = 34% $
Correct Option: B