HCF & LCM Quicknotes

Solve HCF and LCM Problems Quickly

As we know that HCF and LCM is the most underrated topic in mathematics. But it is important to know that the topic consists multiple level of Questions. Let us review thoroughly How To Solve LCM and HCF Quickly.

Highest Common Factor is also known as Greatest Common divisor i.e. the largest positive integer that divides more than one integers called as greatest common divisor.

Least Common Multiple is also known as Smallest Common Multiple i.e. the smallest positive integer that is divisible by more than one integer.

How to Solve HCF as well as LCM Questions Quickly

HCF – It is important to note that HCF of the given numbers cannot be greater than any one of them.
LCM – It is important to note that LCM of the given numbers cannot be smaller than any one of them.
If 1 is the HCF of 2 numbers, then their LCM will be their product.
For two co prime numbers, the HCF is always 1.
The most common methods to solve HCF and LCM easily is

Type 1: Find the greatest or smallest number.

1. The greatest possible length which can be used to measure exactly the lengths 5 m, 4 m, 12 m 55 cm is

Options:

A. 2

B. 10

C. 25

D. 5

Solution:

H.C.F. of (500 cm, 400 cm, 1255 cm) = 5 cm

The factors of 400 are: 1, 2, 4, 5, 8, 10, 16, 20, 25, 40, 50, 80, 100, 200, 400

The factors of 500 are: 1, 2, 4, 5, 10, 20, 25, 50, 100, 125, 250, 500

The factors of 1255 are: 1, 5, 251, 1255

Then the highest common factor is 5

Correct option: D

2. The H.C.F. and L.C.M. of two numbers are 10 and 5040 respectively. If one of the numbers is 175, find the other number?

Options:

A. 288

B. 300

C. 308

D. 280

Solution:

We know that, Product of two numbers = H.C.F x L.C.M

Therefore, a = $\frac{10 × 5040}{175} = \frac{50400}{175} = 288$

So, the other number = 288

Correct option: A

3.Find the greatest number which on dividing 1484 and 2045 leaves remainders 4 and 5 respectively?

Options:

A. 20

B. 30

C. 10

D. 40

Solution:

Required number = H.C.F. of (1484 – 4) and (2045 – 5)

H.C.F. of 1480 and 2040

1480 = 2 x 2 x 2 × 5 × 37

2040 = 2 x 2 x 2 × 3 × 5 × 17

Correct Option: D

Type 2: Find HCF

1. Three numbers are in the ratio of 4: 3: 6 and their L.C.M. are 3600. Find their H.C.F:

Options:

A. 20

B. 30

C. 25

D. 50

Solution:

Let the numbers be 4x, 3x and 6x

Then, their L.C.M. = (4x * 3x *6x) = 72x

So, 72x = 3600 or x = 50

Therefore, numbers are (4 x 50), (3 x 50) and (6 x 50) = 200, 150, 300

The factors of 150 are: 1, 2, 3, 5, 6, 10, 15, 25, 30, 50, 75, 150

The factors of 300 are: 1, 2, 3, 4, 5, 6, 10, 12, 15, 20, 25, 30, 50, 60, 100, 150, 300

The factors of 200 are: 1, 2, 4, 5, 8, 10, 20, 25, 40, 50, 100, 200

Then the highest common factor is 50.

Hence, required H.C.F. = 50

Correct option: D

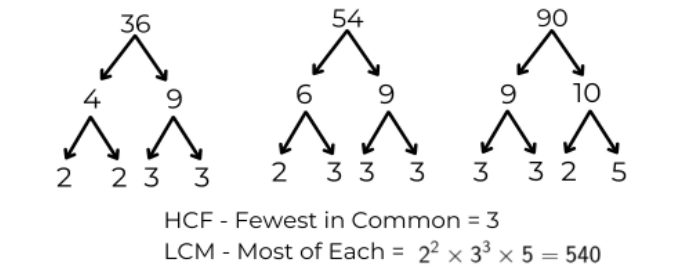
2. Find the HCF of 34, 48, 56, and 74

Options:

A. 2

B. 4

C. 34

D. 8

Solution:

The factors of 34 are: 1, 2, 17, 34

The factors of 48 are: 1, 2, 3, 4, 6, 8, 12, 16, 24, 48

The factors of 56 are: 1, 2, 4, 7, 8, 14, 28, 56

The factors of 74 are: 1, 2, 37, 74

Therefore, the highest common factor is 2.

Correct option: A

3. Find the HCF of $\frac{2}{11},\frac{4}{17},\frac{6}{5}$

Options:

A. $\frac{1}{935}$

B. $\frac{2}{935}$

C. $\frac{2}{93}$

D. $\frac{2}{35}$

Solution:

We know that

HCF = $\frac{HCF\; of\; numerator\;}{LCM\; of\; Denominator\;}$

HCF = $\frac{HCF (2,4,6)}{LCM(11,17,5) }$

HCF = $\frac{2}{935}$

Correct Option: B

Type 3: How to Solve when sum of two numbers is given , LCM and HCF is given to find the sum of reciprocals.

1.Sum of two numbers is 55 and the H.C.F. and L.C.M. of these numbers are 5 and 120 respectively, then the sum of the reciprocals of the numbers is equals to:

Options:

A. $\frac{11}{120}$

B. $\frac{11}{220}$

C. $\frac{21}{120}$

D. $\frac{11}{320}$

Solution:

Let the numbers be a and b.

Let the numbers be a and b.

a × b = HCF × LCM = 5 × 120

HCF × LCM = 600

w, as we know that$ \frac{1}{a} +\frac{1}{b} = \frac{a+b}{a\times b}$

$\frac{1}{a} +\frac{1}{b} = \frac{55}{600}$

$\frac{11}{120}$

Correct option: B

Type 4: How to Solve HCF, LCM Problems related to finding the biggest container to measure quantities

1.Suppose there are three different containers contain different quantities of a mixture of milk and water whose measurements are 403 litres, 434 litres and 465 litres What biggest measure must be there to measure all the different quantities exactly?

Options:

A. 31 litres

B. 21 litres

C. 41 litres

D. 30 litres

Solution:

Prime factorization of 403,434 and 465 is

403=13×31

434=2×7×31

465=3×5×31

H.C.F of 403, 434 and 465=31

Correct option: A

Type 5: How to Solve HCF, LCM Problems related to Bell ring.

1. Six bells commence tolling together and toll at intervals of 2, 4, 6, 8 10 and 12 seconds respectively. In 30 minutes, how many times do they toll together ?

Options:

A. 8

B. 16

C. 9

D. 10

Solution:

L.C.M. of 2, 4, 6, 8, 10, 12 is 120.

Hence, the bells will toll together after every 120 seconds(2 minutes).

Therefore, in 30 minutes ,number of times bells toll together is $\frac{30}{2} + 1$ = 16

Correct Option: B

Type 6 :How to Solve HCF, LCM Problems related to Circle Based Runner

1. Two people P and Q start running towards a circular track of length 400 m in opposite directions with initial speeds of 10 m/s and 40 m/s respectively. Whenever they meet, P’s speed doubles and Q’s speed halves. After what time from the start will they meet for the third time?

Options:

A. 30 seconds

B. 26 seconds

C. 10 seconds

D. 20 seconds

Solution:

Time taken to meet for the 1st time = $\frac{400}{40+10} = 8 $sec.

Time taken to meet for the 2nd time = $\frac{400}{20+20}$ = 10 sec.

Now P’s speed =40 m/sec and Q’s speed = 10 m/sec.

Time taken to meet for the 3rd time = $\frac{400}{10+40}$

Therefore, Total time= (8+10+8) = 26 seconds.

Correct Option: B

Courses

Chapters

Aptitude

HCF & LCM Quicknotes