Simple and Compound Interest Tip and Tricks

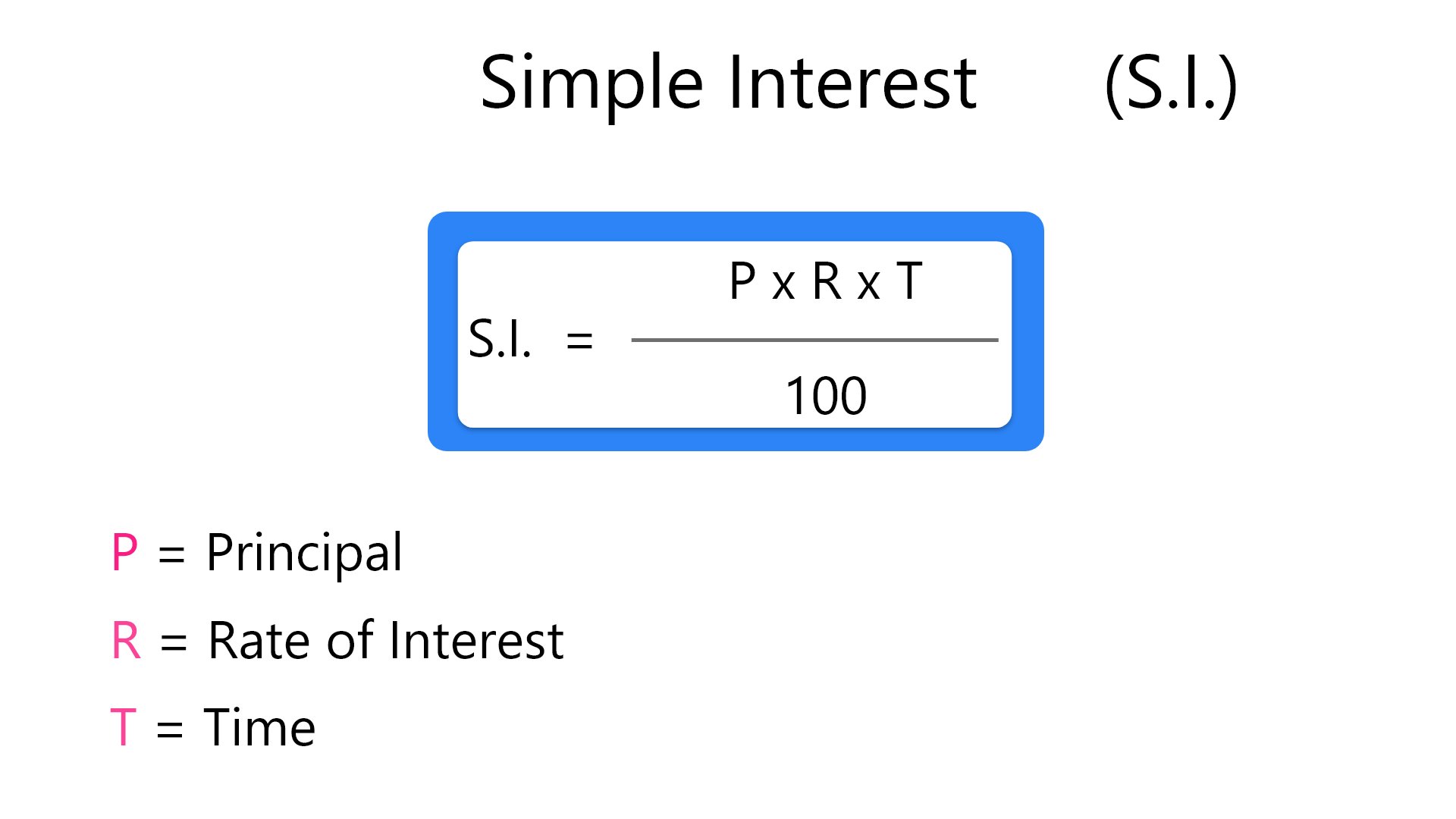
• Simple interest is calculated by multiplying the interest rate and principal and number of days.
• Here, are quick and easy tips and tricks on Simple Interest problems learn easily tricks and tips on SI and efficiently in competitive exams and recruitment drive process.
• There are mainly 2 types of questions asked in exams. However, these questions can be twisted to check the students' understanding level of the topic.

• If the interest on a sum of money is $\frac{1}{x}$ of the principal, and the number of years is equal to the rate of interest then rate can be calculated using the formula: $ \sqrt{\frac{100}{x}}$ .

• The rate of interest for $t_1$ years is $r_1%, t_2$ years is $r_2%, t_3$ years is $r_3 \%.$ If a man gets interest of Rs x for $(t_1+ t_2 + t_3 = n)$ years, then principal is given by: $ \frac{x \times 100}{r_1 \times t_1} + r_2 \times t_2 + r_3 \times t_3 $

• If sum of money becomes x times in t years at simple interest, then the rate is calculated as $ R = \frac{100 (x - 1)}{t}\% $

• If a sum of money becomes x times in t years at simple rate of interest, then the time is calculated as $ t = \frac{100 (x - 1)}{R}\% $

• If an amount $P_1$ is lent out at simple interest of $R_1\%$ p.a. and another amount of $P_2$ at simple interest of $R_2\%$ p.a, then the rate of interest of the whole sum is given by: $ R = \frac{{P_1}{R_1} + {P_2}{R_2}}{P_1 + P_2}$

Question 1. Find the simple interest of Rs 1000 for 10 years at 5% p.a.?

Options:

A. Rs. 5500

B. Rs. 6000

C. Rs. 5000

D. Rs. 1000

Solution :

We know that,

$SI = \frac{p \times r \times t}{100}$

$SI = \frac{1000 \times 5 \times 10}{100}$

$SI = \frac{50000}{100}$

$SI = Rs. 500$

Correct option: C

Question 1. If a sum of Rs. 25000 is given as loan for a period of 4 years with the interest rates 2%, 4%, 5%, and 6% for the $1^{st}, 2^{nd}, 3^{rd},$ and $4^{th}$ year respectively. What is the total amount that has to be paid at the end of 4 years?

Options:

A. Rs. 29250

B. Rs. 29520

C. Rs. 25920

D. Rs. 25290

Solution :

As this is a case of Simple Interest we add 2% + 4% + 5% and 6% = 17%.

Amount = Principal + Rate of Interest.

Principal is 100% of the amount.

Therefore,

Amount = 100% + 17%.

Therefore Amount = 117%

Amount = $ \frac{(117 \times 25000)}{100}$

= 29250

Correct option: A

Question 1. A sum of money becomes 5 times in 20 years. Calculate the rate of interest.

Options:

A. 20%

B. 13%

C. 15%

D. 25%

Solution :

We know that, If sum of money becomes x times in t years at simple interest, then the rate is calculated as

$R = 100 \frac{x-1}{t} %$

$R = 100 \frac{5-1}{20}$

$R = 100 \frac{4}{20}$

$R = \frac{400}{20}$

$R = 20\%$

Correct option: A

Compound interest is the interest calculated on the initial principal and also includes all the interest of previous periods on a deposit or loan. Here we can find Tips Tricks And Shortcuts Of Compound Interest.

Compound Interest can be calculated , CI = P $ ( 1 + {\frac{r}{100n}})^{nt} - P$

Total Amount can be calculated, A = P $ ( 1 + {\frac{r}{100n}})^{nt}$

A sum of money placed at compound interest becomes x time in ‘a’ years and y times in ‘b’ years. These two sums can be related by the following formula: $x^{\frac{1}{a}} = y^{\frac{1}{b}}$

If an amount of money grows up to Rs x in t years and up to Rs y in (t+1) years on compound interest, then $ r\% = \frac{y-x}{x} \times 100$

A sum at a rate of interest compounded yearly becomes Rs. $A_1$ in t years and Rs. $A_2$ in (t + 1) years, then $ P = A_1(\frac{A_1}{A_2})$

Question 1. Find the amount if Rs 50000 is invested at 10% p.a. for 5 years

Options:

A. 88205

B. 7420.5

C. 80525.5

D. 78502.5

Solution :

We know that,

$Amount = P ( 1 + \frac{r}{100})^{n}\%$

$A = P ( 1 + \frac{10}{100})^{5}\%$

$A = 50000 (1.1)^{5}\%$

$A = 80525.5$

Correct option: C

Question 1. The Compound Interest on a sum of Rs 576 is Rs 100 in two years. Find the rate of interest.

Options:

A. 7.33%

B. 4.33%

C. 8.33%

D. 5.33%

Solution :

We know that,

Amount = Compound Interest + Principal

$\implies A = 576 + 100 = 676$

$\implies A = P ( 1 + \frac{r}{100})^{n}\%$

$\implies 676 = 576 ( 1 + \frac{r}{100})^{2}\%$

$\implies \frac{676}{576} = ( 1 + \frac{r}{100})^{2}\%$

$\implies (\frac{26}{24})^2 = ( 1 + \frac{r}{100})\%$

$\implies (\frac{26}{24})= ( 1 + \frac{r}{100})\%$

$\implies 1.0833 - 1 = (\frac{r}{100})\%$

$\implies 0.0833= (\frac{r}{100})\%$

$\implies r = 8.33\%$

Correct option: C

Question 1. The difference between the CI and SI on a certain amount at 14% p.a. for 2 years is Rs. 100. What will be the value of the amount at the end of three years if compounded annually?

Options:

A. 7558.89

B. 7500.45

C. 7558

D. 7554.56

Solution :

We know that, Difference between CI and SI for 2 years , Difference = $\frac{P(R)^2}{100^2}$

$100 = \frac{P(14)^2}{100^2}$

$\implies P = \frac{100(100)^2}{14^2}$

$\implies P = \frac{1000000}{196}$

$\implies P = 5102.04$

Now calculate the CI on Rs. 5102.04

$\implies A = P ( 1 + \frac{r}{100})^{n}$

$\implies A = 5102.04 ( 1 + \frac{14}{100})^{3}$

$\implies A = 5102.04 \times 1.48 $

$\implies A = 7558.89 $

Correct option: A

Question 1. A sum of money placed at compound interest doubles itself in 8 years. In how many years will it amount to 32 times itself?

Options:

A. 45 years

B. 60 years

C. 50 years

D. 40 years

Solution :

We know that,

$x^{\frac{1}{a}} = y\frac{1}{b}$

$2^{\frac{1}{8}} = 32\frac{1}{x}$

$2^{\frac{1}{8}} = 2\frac{5}{x}$

$\frac{1}{8} = \frac{5}{x}$

$ x = 40 years$

Correct option: D

Question 1. Find the compound interest on a sum of money of Rs.10000 after 2 years, if the rate of interest is 2% for the first year and 4% for the next year ?

Options:

A. Rs. 304

B. Rs. 608

C. Rs. 1000

D. Rs. 710

Solution :

We know that,

$Amount = P (1 + \frac{r_1}{100})(1 + \frac{r_2}{100})(1 + \frac{r_3}{100})$

$\text{Here,} r_1 = 2\% r_2 = 4\%$ and $ P = Rs. 10000,$

$CI = A - P$

$CI = 10000 (1 + \frac{2}{100})(1 + \frac{4}{100}) - 10000$

$CI = 10000 (\frac{102}{100})(\frac{104}{100}) - 10000$

$CI = 10000 (\frac{2652}{2500}) - 10000$

$CI = 10000 \times 1.06 - 10000$

$CI = 10608 - 10000$

$CI = 608$

Correct option: B